A randomized singular value decomposition for third-order oriented tensors

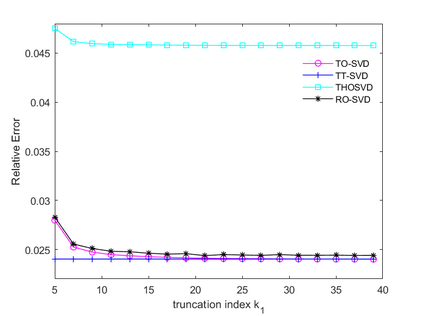
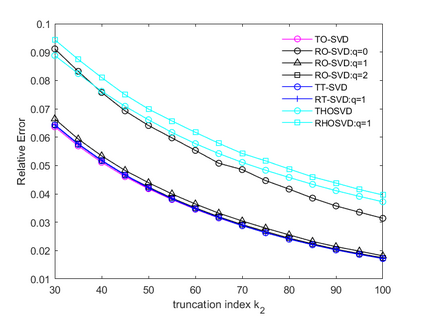
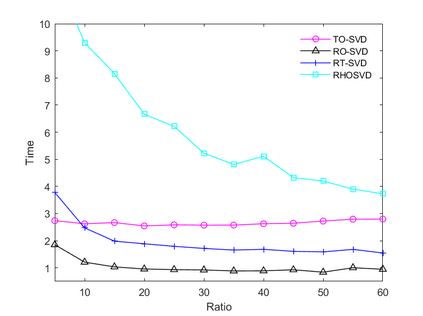
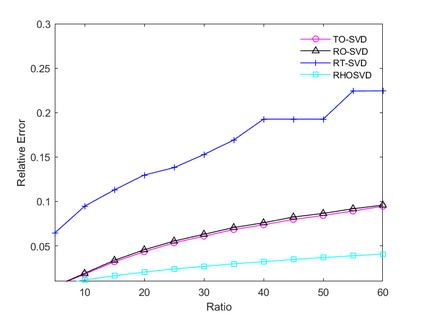
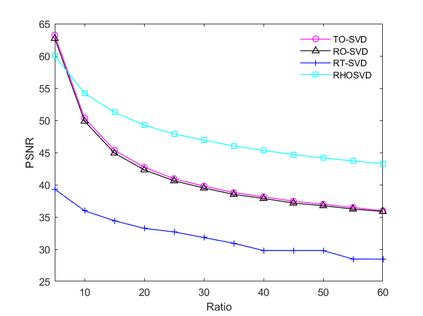
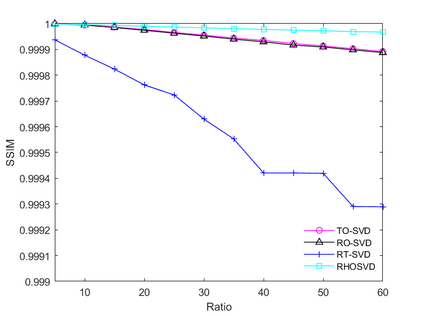
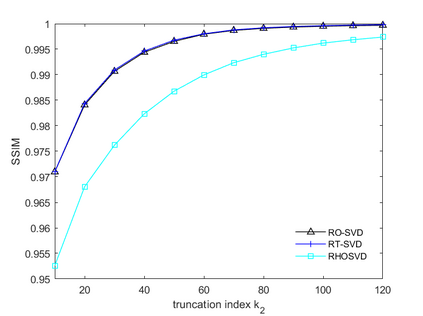
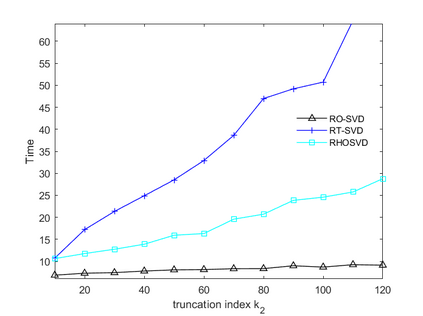
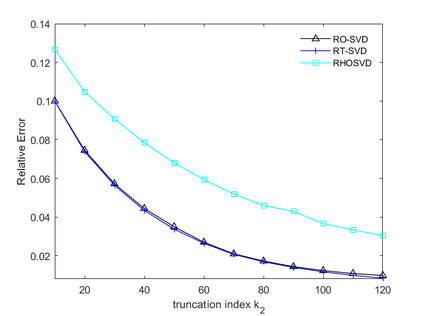
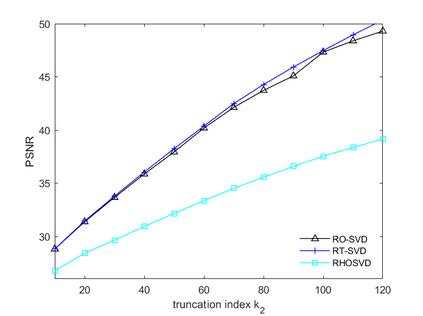
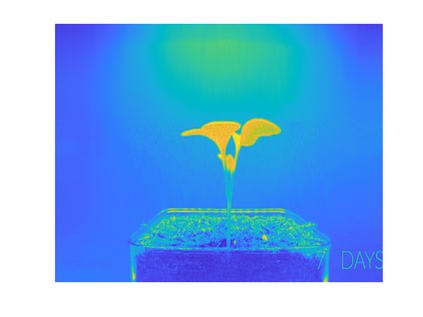
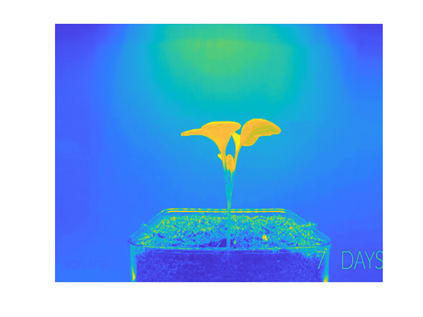
The oriented singular value decomposition (O-SVD) proposed in [Numer. Linear Algebra Appl., 27(2020), e2290] provides a hybrid approach to the t-product based third-order tensor singular value decomposition with the transform matrix being a factor matrix of the higher order singular value decomposition. Continuing along this vein, this paper explores realizing the O-SVD more efficiently by the tensor-train rank-1 decomposition and gives a truncated O-SVD. Motivated by the success of probabilistic algorithms, we develop a randomized version of the O-SVD and present its detailed error analysis. The new algorithm has advantages in efficiency while keeping good accuracy compared with the current tensor decompositions. Our claims are supported by numerical experiments on several oriented tensors from real applications.