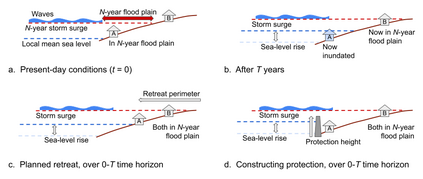
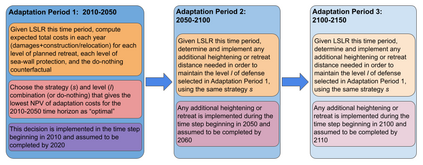
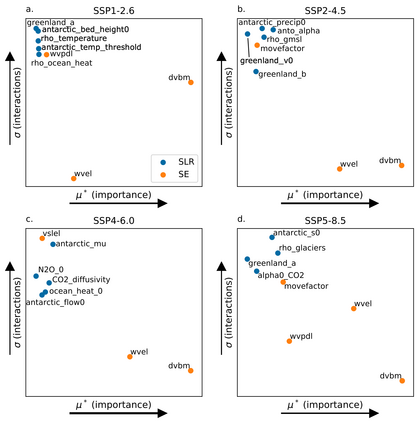
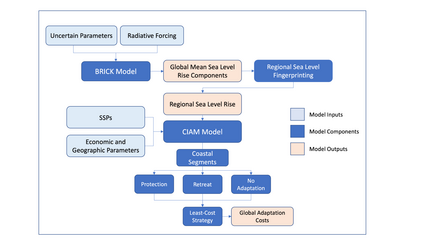
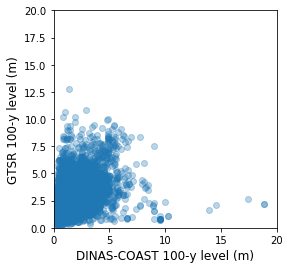
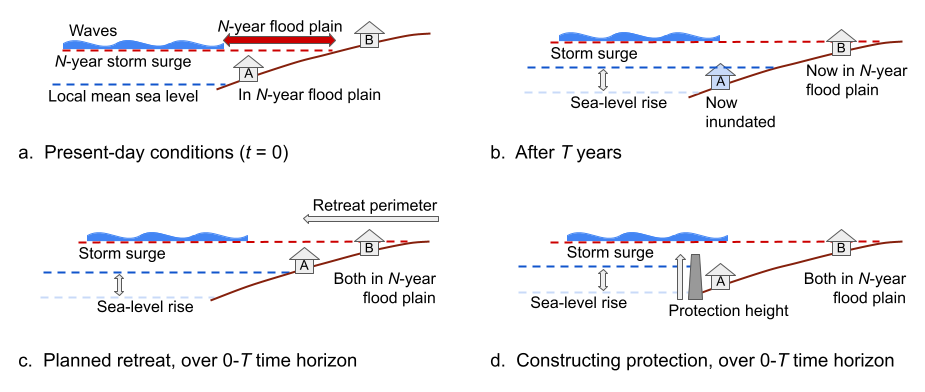
Sea-level rise and associated flood hazards pose severe risks to the millions of people globally living in coastal zones. Models representing coastal adaptation and impacts are important tools to inform the design of strategies to manage these risks. Representing the often deep uncertainties influencing these risks poses nontrivial challenges. A common uncertainty characterization approach is to use a few benchmark cases to represent the range and relative probabilities of the set of possible outcomes. This has been done in coastal adaptation studies, for example, by using low, moderate, and high percentiles of an input of interest, like sea-level changes. A key consideration is how this simplified characterization of uncertainty influences the distributions of estimated coastal impacts. Here, we show that using only a few benchmark percentiles to represent uncertainty in future sea-level change can lead to overconfident projections and underestimate high-end risks as compared to using full ensembles for sea-level change and socioeconomic parametric uncertainties. When uncertainty in future sea level is characterized by low, moderate, and high percentiles of global mean sea-level rise, estimates of high-end (95th percentile) damages are underestimated by between 18% (SSP1-2.6) and 46% (SSP5-8.5). Additionally, using the 5th and 95th percentiles of sea-level scenarios underestimates the 5-95% width of the distribution of adaptation costs by a factor ranging from about two to four, depending on SSP-RCP pathway. The resulting underestimation of the uncertainty range in adaptation costs can bias adaptation and mitigation decision-making.
翻译:代表沿海适应和影响模型是指导制定管理这些风险的战略的重要工具。代表着影响这些风险的往往十分深刻的不确定性,提出了非三重挑战。一种常见的不确定性定性方法是使用几个基准案例来代表一套可能结果的范围和相对概率。这是在沿海适应研究中进行的,例如,使用低、中和高百分位的感兴趣投入,如海平面变化。一个关键的考虑因素是,这种对不确定性的简化定性是如何影响估计沿海影响沿海影响分布的。在这里,我们表明,仅使用几个基准百分位来代表未来海平面变化的不确定性,可能会导致过于自信的预测,并低估高端风险,而与使用全方位的海平面变化和社会经济参数不确定性相比。当未来海平面不确定性的特征是低、中和高百分位的,如海平面变化。当全球平均海平面升高,对高端(95%)的不确定性估计会影响估计沿海影响估计影响的分布。我们表明,仅使用几个基准百分位数来代表未来海平面变化的不确定性的不确定性,而采用S-2/25度调整幅度的汇率的不确定性的预测值成本为18(S-S-S-S-25-95)的海平比值的海平面平面的汇率的调整成本的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的估值的数值为5-比例为5-比例为5-比例为5-比例为5至85的数值为5至5至85的幅度。