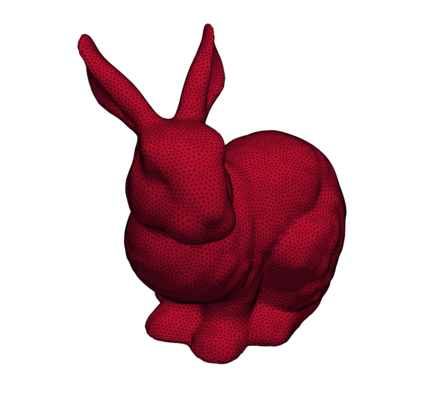
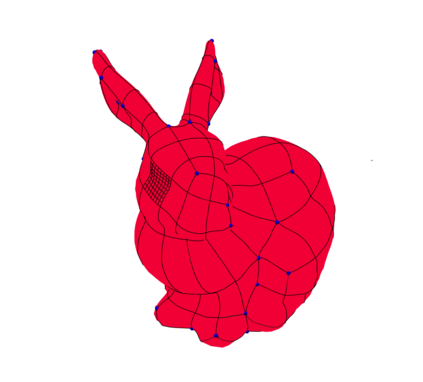
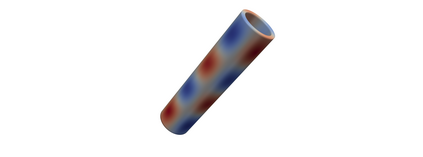
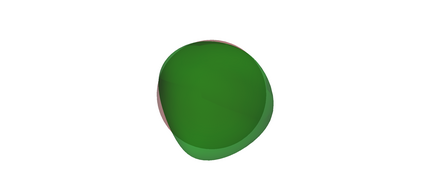
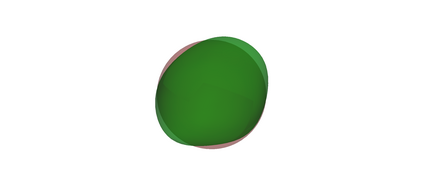
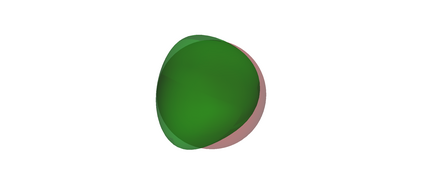
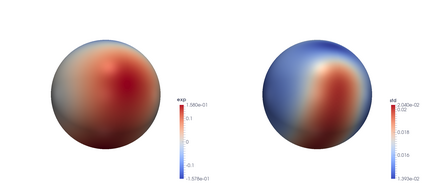
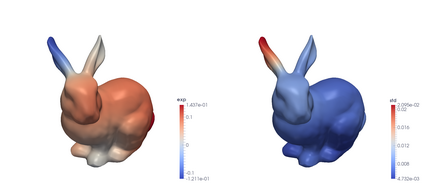
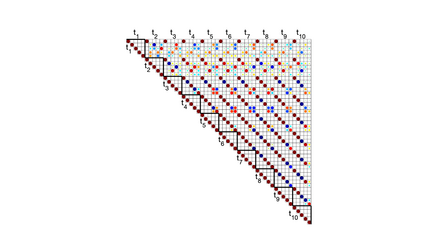
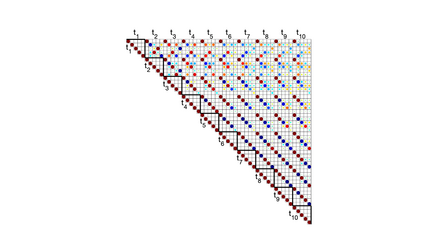
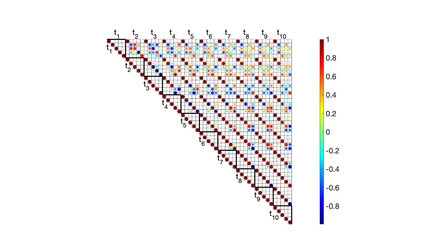
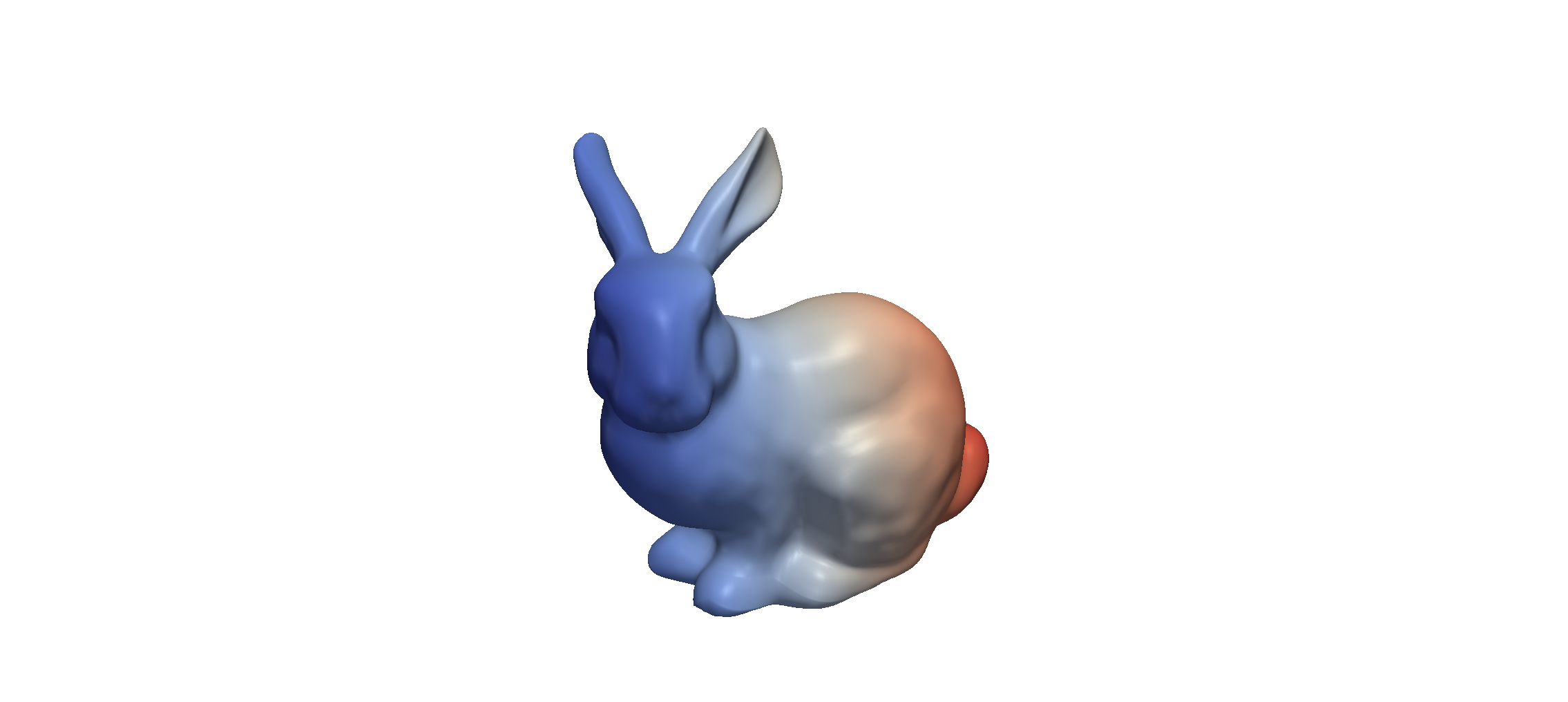
In this article, we discuss the numerical solution of diffusion equations on random surfaces within the isogeometric framework. Complex computational geometries, given only by surface triangulations, are recast into the isogeometric context by transforming them into quadrangulations and a subsequent interpolation procedure. Moreover, we describe in detail, how diffusion problems on random surfaces can be modelled and how quantities of interest may be derived. In particular, we propose a low rank approximation algorithm for the high-dimensional space-time correlation of the random solution. Extensive numerical studies are provided to quantify and validate the approach.
翻译:在本篇文章中,我们讨论了在等离子测量框架内随机表面扩散方程式的数值解决方案。只有地表三角方程给出的复杂计算方程,通过将其转换成四面形和随后的内插程序,被重新纳入等离子测量环境。此外,我们详细描述了如何模拟随机表面扩散问题以及如何得出利息的数量。特别是,我们建议为随机解决方案的高维空间-时间相关性采用低级近似算法。我们提供了广泛的数字研究,以量化和验证该方法。