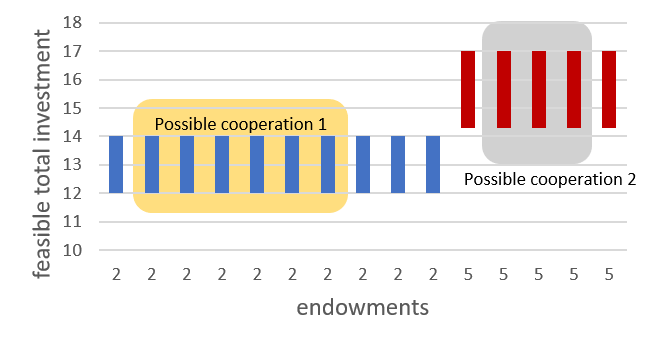
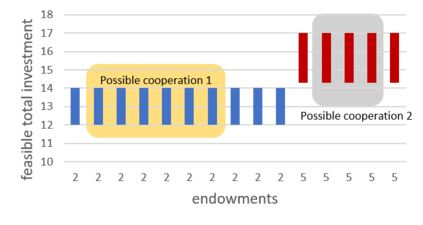
When can cooperation arise from self-interested decisions in public goods games? And how can we help agents to act cooperatively? We examine these classical questions in a pivotal participation game, a variant of public good games, where heterogeneous agents make binary participation decisions on contributing their endowments, and the public project succeeds when it has enough contributions. We prove it is NP-complete to decide the existence of a cooperative Nash equilibrium such that the project succeeds. We also identify two natural special scenarios where this decision problem is tractable. We then propose two algorithms to help cooperation in the game. Our first algorithm adds an external investment to the public project, and our second algorithm uses matching funds. We show that the cost to induce a cooperative Nash equilibrium is near-optimal for both algorithms. Finally, the cost of matching funds can always be smaller than the cost of adding an external investment. Intuitively, matching funds provide a greater incentive for cooperation than adding an external investment does.
翻译:什么时候合作能产生于公益游戏中自利的决定? 以及我们如何帮助代理商合作行动? 我们在一个关键的参与游戏中研究这些古典问题,一个公益游戏的变种,在这个游戏中,各种代理商在贡献其天赋时作出二进制参与决定,而公共项目在有足够贡献时成功。我们证明,决定是否存在合作型纳什平衡,从而让项目成功,是NP不完整的。我们还确定了两种自然的特殊情形,即决定问题可以引起。我们然后提出两种算法,以帮助在游戏中开展合作。我们的第一个算法为公共项目增加了外部投资,而我们的第二个算法则使用匹配资金。我们表明,激励合作型纳什平衡的成本几乎是两种算法的最佳选择。最后,匹配基金的成本总是比增加外部投资的成本要低。直觉地说,匹配资金比增加外部投资更能激励合作。