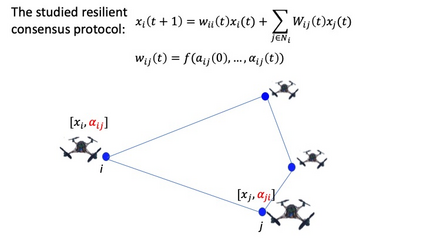
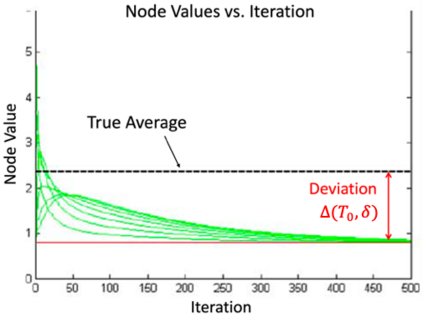
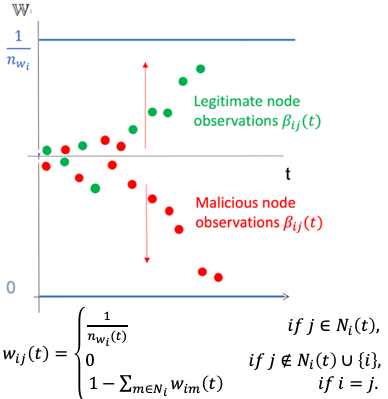
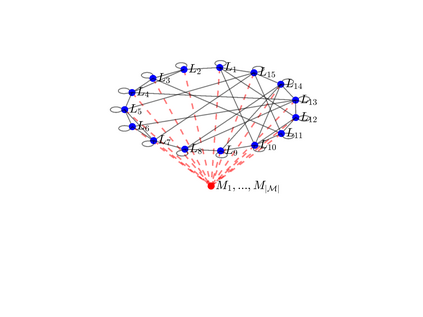
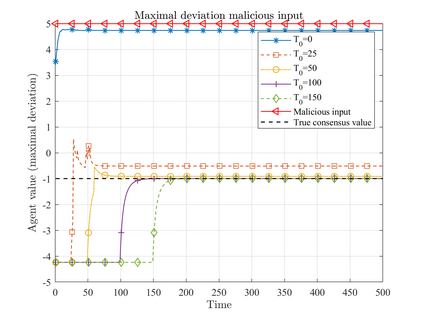
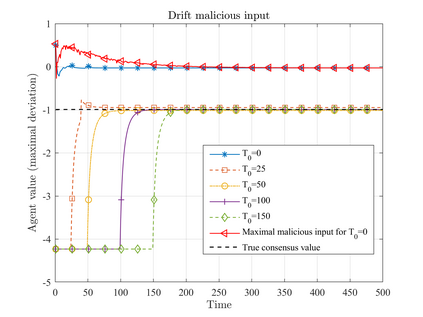
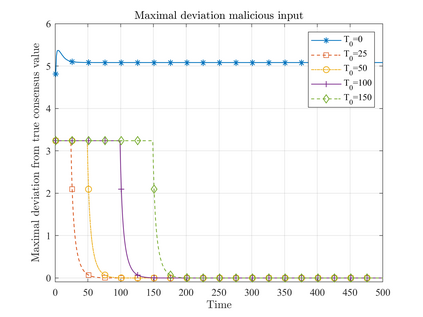
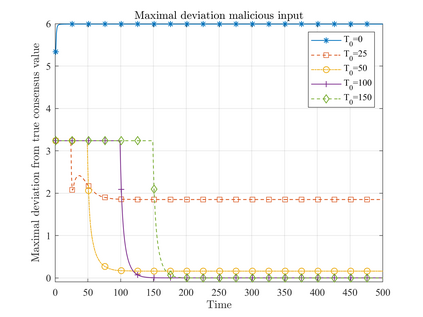
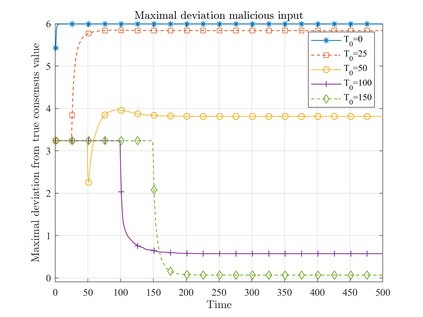
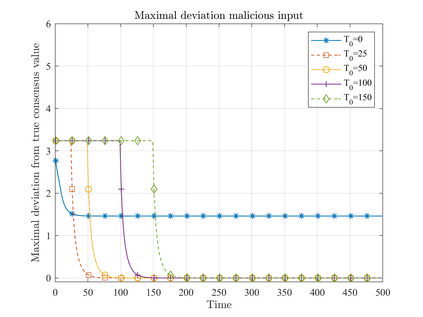
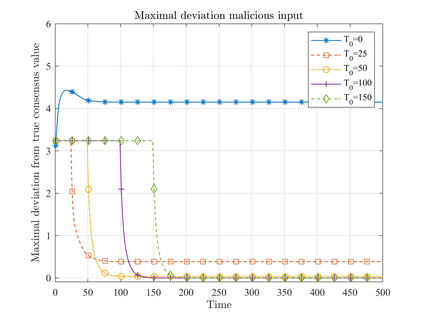
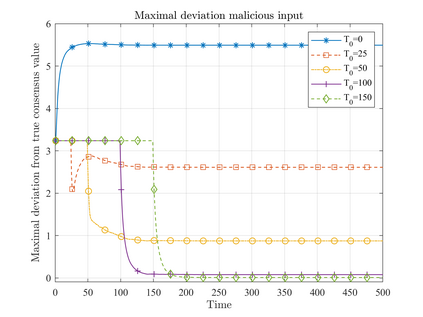
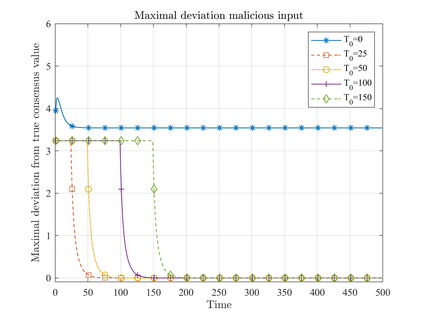
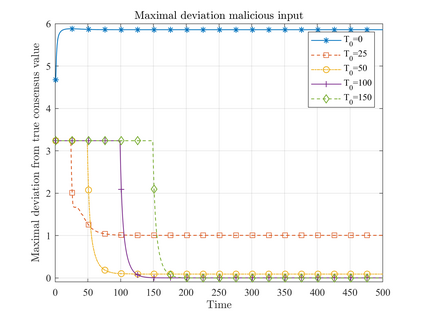
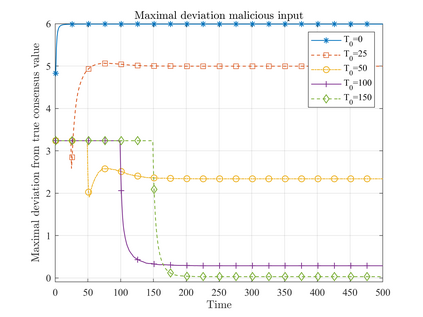
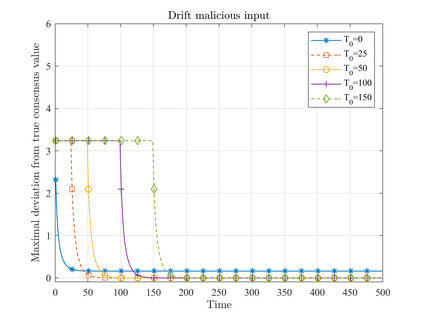
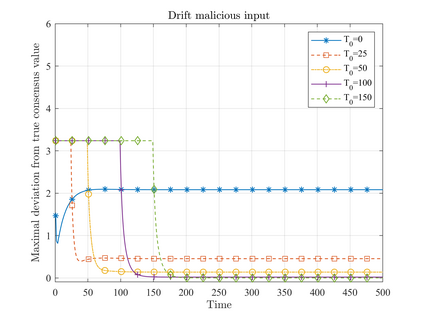
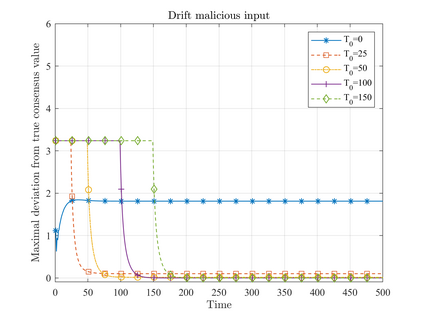
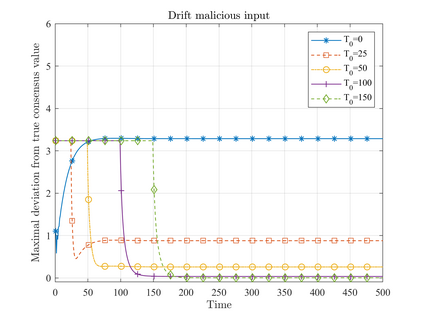
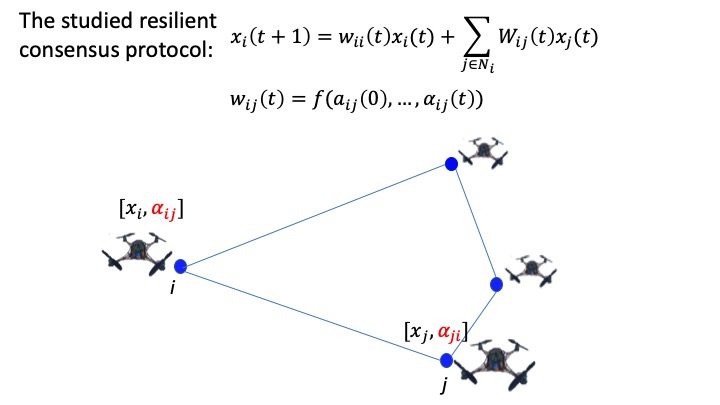
This work considers the problem of resilient consensus where stochastic values of trust between agents are available. Specifically, we derive a unified mathematical framework to characterize convergence, deviation of the consensus from the true consensus value, and expected convergence rate, when there exists additional information of trust between agents. We show that under certain conditions on the stochastic trust values and consensus protocol: 1) almost sure convergence to a common limit value is possible even when malicious agents constitute more than half of the network connectivity, 2) the deviation of the converged limit, from the case where there is no attack, i.e., the true consensus value, can be bounded with probability that approaches 1 exponentially, and 3) correct classification of malicious and legitimate agents can be attained in finite time almost surely. Further, the expected convergence rate decays exponentially with the quality of the trust observations between agents.
翻译:这项工作考虑的是具有弹性的共识问题,其中代理商之间的信任价值是具有弹性的。具体地说,当代理商之间有额外的信任信息时,我们得出一个统一的数学框架,以描述趋同、偏离共识的真正共识价值和预期的趋同率。我们表明,在某些条件下,在代理商之间的信任价值和共识协议上,我们表明:(1) 几乎可以肯定,即使恶意代理商占网络连通性一半以上的情况下,也有可能达到共同限值;(2) 趋同限度的偏离,从没有攻击的情况(即真正的共识值)看,可以与下述可能性相约束:即接近1的概率指数化;(3) 对恶意和合法代理商的正确分类几乎可以肯定地在有限时间内达到。此外,预期的趋同率随着代理商之间信任观察的质量而急剧下降。