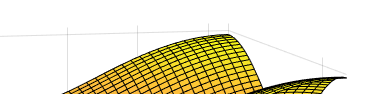
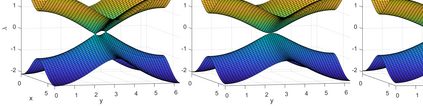
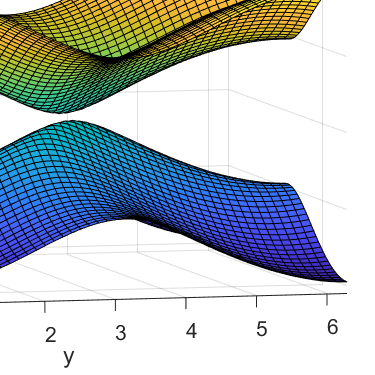
A simple iterative scheme is proposed for locating the parameter values for which a 2-parameter family of real symmetric matrices has a double eigenvalue. The convergence is proved to be quadratic. An extension of the scheme to complex Hermitian matrices (with 3 parameters) and to location of triple eigenvalues (5 parameters for real symmetric matrices) is also described. Algorithm convergence is illustrated in several examples: a real symmetric family, a complex Hermitian family, a family of matrices with an "avoided crossing" (no covergence) and a 5-parameter family of real symmetric matrices with a triple eigenvalue.
翻译:提议了一个简单的迭代办法,用于确定实际对称矩阵的2参数组具有双重等值的参数值。这种组合被证明是二次组合。这个办法扩大到复杂的Hermitian矩阵(有3个参数)和3个对称矩阵(有5个实际对称矩阵参数)的位置。Agorithm趋同在若干例子中作了说明:一个真正的对称组合、一个复杂的Hermitian家族、一个具有“避免交叉”的矩阵组(没有隐蔽性)和一个具有3个埃米提矩阵的5个实际对称矩阵组(有3个对称矩阵值)。
相关内容
- Today (iOS and OS X): widgets for the Today view of Notification Center
- Share (iOS and OS X): post content to web services or share content with others
- Actions (iOS and OS X): app extensions to view or manipulate inside another app
- Photo Editing (iOS): edit a photo or video in Apple's Photos app with extensions from a third-party apps
- Finder Sync (OS X): remote file storage in the Finder with support for Finder content annotation
- Storage Provider (iOS): an interface between files inside an app and other apps on a user's device
- Custom Keyboard (iOS): system-wide alternative keyboards
Source: iOS 8 Extensions: Apple’s Plan for a Powerful App Ecosystem