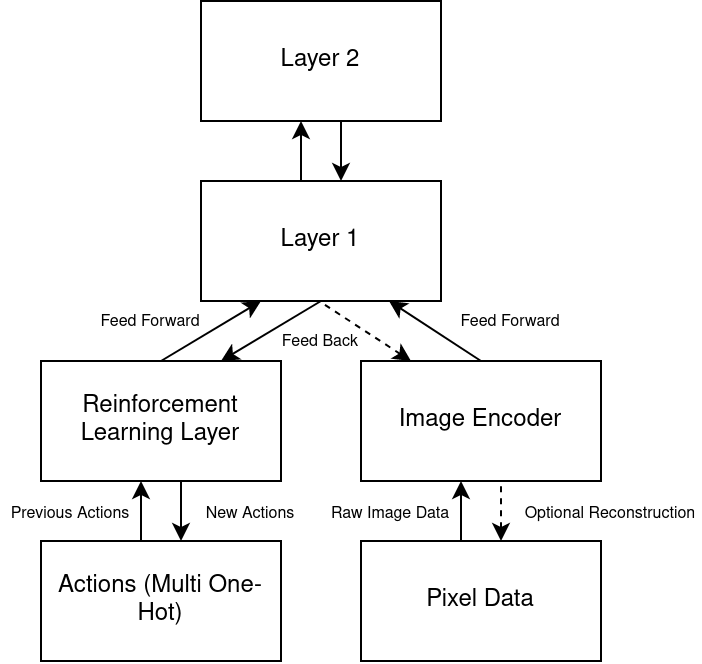
不需要借助GPU的力量，用树莓派也能实时训练agent玩Atari

机器之心报道

参与：Racoon X

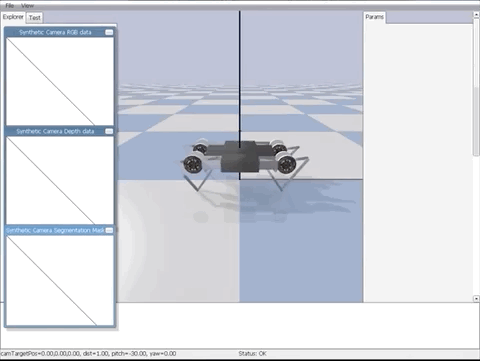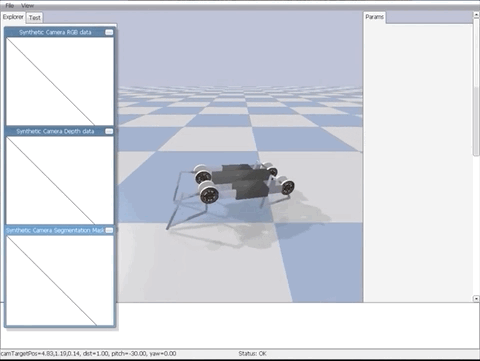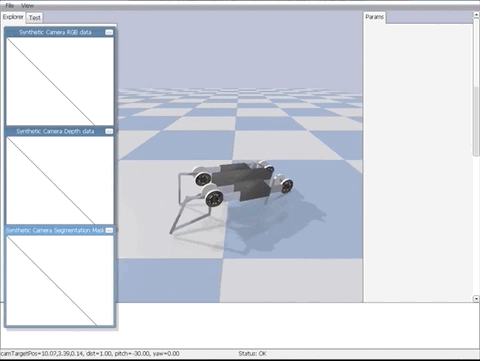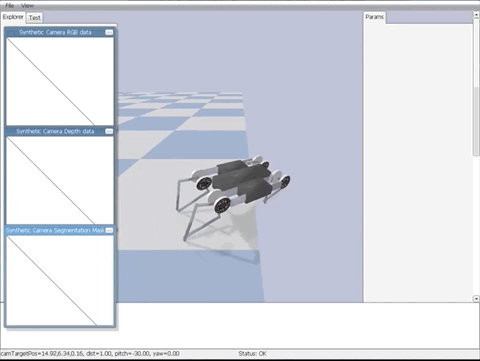
还是熟悉的树莓派！训练 RL agent 打 Atari 不再需要 GPU 集群，这个项目让你在边缘设备上也能进行实时训练。

自从 DeepMind 团队提出 DQN，在 Atari 游戏中表现出超人技巧，已经过去很长一段时间了。在此期间持续有新的方法被提出，不断创造出 Deep RL 领域新 SOTA。然而，目前不论是同策略或异策略强化学习方法（此处仅比较无模型 RL），仍然需要强大的算力予以支撑。即便研究者已将 Atari 游戏的分辨率降低到 84x84，一般情况下仍然需要使用 GPU 进行策略的训练。

如今，来自 Ogma Intelligent Systems Corp. 的研究人员突破了这一限制。他们在稀疏预测性阶层机制（Sparse Predictive Hierarchies）的基础上，提出一种不需要反传机制的策略搜索框架，使得实时在树莓派上训练 Atari 游戏的控制策略成为可能。下图展示了使用该算法在树莓派上进行实时训练的情形。

可以看到，agent 学会了如何正确调整滑块位置来接住小球，并发动进攻的策略。值得注意的是，观测输入为每一时刻产生的图片。

也就是说，该算法做到了在树莓派这样算力较小的边缘设备上，实时学习从像素到策略的映射关系。

研究者开源了他们的 SPH 机制实现代码，并提供了相应 Python API。这是一个结合了动态系统应用数学、计算神经科学以及机器学习的扩展库。他们的方法曾经还被 MIT 科技评论列为「Best of the Physics arXiv」。

项目地址：

https://github.com/ogmacorp/OgmaNeo2

OgmaNeo2

研究者所提出的 SPH 机制不仅在 Pong 中表现良好，在连续策略领域也有不错的表现。下图分别是使用该算法在 OpenAI gym 中 Lunar Lander 环境与 PyBullet 中四足机器人环境的训练结果。

在 Lunar Lander 环境中，训练 1000 代之后，每个 episode 下 agent 取得了平均 100 分左右的 reward。如果训练时间更长（3000 代以上），agent 的平均 reward 甚至能达到 200。在 PyBullet 的 Minitaur 环境中，agent 的训练目标是在其自身能量限制条件下，跑得越快越好。从图中可以看到，经过一段时间训练，这个四足机器人学会了保持身体平衡与快速奔跑（虽然它的步态看起来不是那么地自然）。看起来效果还是很棒的，机器之心也上手测试了一番。

算法框架

OgmaNeo2 用来学习 Pong 控制策略的整体框架如下图所示。图像观测值通过图像编码器输入两层 exponential memory 结构中，计算结果输出到之后的 RL 层产生相应动作策略。

项目实测

在安装 PyOgmaNeo2 之前，我们需要先编译安装其对应的 C++库。将 OgmaNeo2 克隆到本地：

!git clone https://github.com/ogmacorp/OgmaNeo2.git

之后将工作目录切换到 OgmaNeo2 下，并在其中创建一个名为 build 的文件夹，用于存放编译过程产生的文件。

import os
os.chdir('OgmaNeo2')
!mkdir build
os.chdir('build')

接下来我们对 OgmaNeo2 进行编译。这里值得注意的是，我们需要将-DBUILD_SHARED_LIBS=ON 命令传入 cmake 中，这样我们才能在之后的 PyOgmaNeo2 扩展库里使用它。

!cmake .. -DBUILD_SHARED_LIBS=ON
!make
!make install

当 OgmaNeo2 安装成功后，安装 SWIG v3 及 OgmaNeo2 的相应 Python 扩展库：

!apt-get install swig3.0
os.chdir('/content')
!git clone https://github.com/ogmacorp/PyOgmaNeo2
os.chdir('PyOgmaNeo2')
!python3 setup.py install --user

接下来输入 import pyogmaneo，如果没有错误提示就说明已经成功安装了 PyOgmaNeo2。

我们先用一个官方提供的时间序列回归来测试一下，在 notebook 中输入：

import numpy as np
import pyogmaneo
import matplotlib.pyplot as plt
# Set the number of threads
pyogmaneo.ComputeSystem.setNumThreads(4)
# Create the compute system
cs = pyogmaneo.ComputeSystem()
# This defines the resolution of the input encoding - we are using a simple single column that represents a bounded scalar through a one-hot encoding. This value is the number of "bins"
inputColumnSize = 64
# The bounds of the scalar we are encoding (low, high)
bounds = (-1.0, 1.0)
# Define layer descriptors: Parameters of each layer upon creation
lds = []
for i in range(5): # Layers with exponential memory
    ld = pyogmaneo.LayerDesc()
    # Set the hidden (encoder) layer size: width x height x columnSize
    ld.hiddenSize = pyogmaneo.Int3(4, 4, 16)
    ld.ffRadius = 2 # Sparse coder radius onto visible layers
    ld.pRadius = 2 # Predictor radius onto sparse coder hidden layer (and feed back)
    ld.ticksPerUpdate = 2 # How many ticks before a layer updates (compared to previous layer) - clock speed for exponential memory
    ld.temporalHorizon = 2 # Memory horizon of the layer. Must be greater or equal to ticksPerUpdate, usually equal (minimum required)

    lds.append(ld)
# Create the hierarchy: Provided with input layer sizes (a single column in this case), and input types (a single predicted layer)
h = pyogmaneo.Hierarchy(cs, [ pyogmaneo.Int3(1, 1, inputColumnSize) ], [ pyogmaneo.inputTypePrediction ], lds)
# Present the wave sequence for some timesteps
iters = 2000
for t in range(iters):
    # The value to encode into the input column
    valueToEncode = np.sin(t * 0.02 * 2.0 * np.pi) * np.sin(t * 0.035 * 2.0 * np.pi + 0.45) # Some wavy line
    valueToEncodeBinned = int((valueToEncode - bounds[0]) / (bounds[1] - bounds[0]) * (inputColumnSize - 1) + 0.5)
    # Step the hierarchy given the inputs (just one here)
    h.step(cs, [ [ valueToEncodeBinned ] ], True) # True for enabling learning
    # Print progress
    if t % 100 == 0:
        print(t)
# Recall the sequence
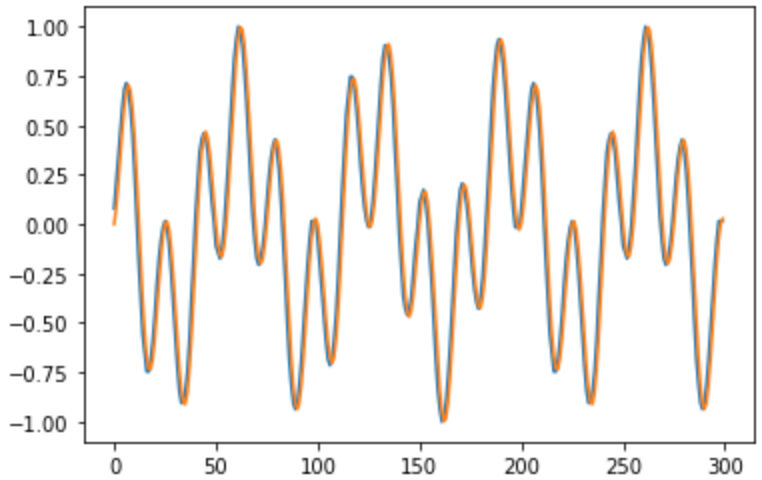
ts = [] # Time step
vs = [] # Predicted value
trgs = [] # True value
for t2 in range(300):
    t = t2 + iters # Continue where previous sequence left off
    # New, continued value for comparison to what the hierarchy predicts
    valueToEncode = np.sin(t * 0.02 * 2.0 * np.pi) * np.sin(t * 0.035 * 2.0 * np.pi + 0.45) # Some wavy line
    # Bin the value into the column and write into the input buffer. We are simply rounding to the nearest integer location to "bin" the scalar into the column
    valueToEncodeBinned = int((valueToEncode - bounds[0]) / (bounds[1] - bounds[0]) * (inputColumnSize - 1) + 0.5)
    # Run off of own predictions with learning disabled
    h.step(cs, [ [ valueToEncodeBinned ] ], False) # Learning disabled
    predIndex = h.getPredictionCs(0)[0] # First (only in this case) input layer prediction

    # Decode value (de-bin)
    value = predIndex / float(inputColumnSize - 1) * (bounds[1] - bounds[0]) + bounds[0]
    # Append to plot data
    ts.append(t2)
    vs.append(value)
    trgs.append(valueToEncode)
    # Show predicted value
    print(value)
# Show plot
plt.plot(ts, vs, ts, trgs)

可得到如下结果。图中橙色曲线为真实值，蓝色曲线为预测值。可以看到，该方法以极小的误差拟合了真实曲线。

最后是该项目在 CartPole 任务中的表现。运行!python3 ./examples/CartPole.py，得到如下训练结果。可以看到，其仅用 150 个 episode 左右即解决了 CartPole 任务。

本文为机器之心报道，转载请联系本公众号获得授权。

✄------------------------------------------------

加入机器之心（全职记者 / 实习生）：hr@jiqizhixin.com

投稿或寻求报道：content @jiqizhixin.com

广告 & 商务合作：bd@jiqizhixin.com