A flexible class of priors for conducting posterior inference on structured orthonormal matrices

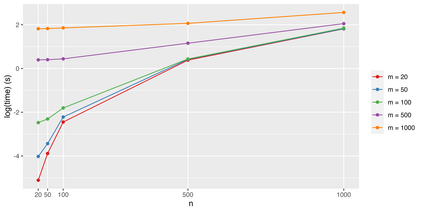
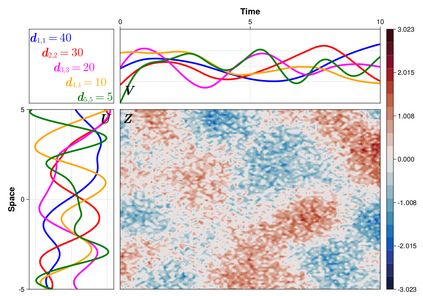
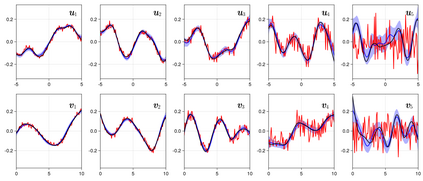
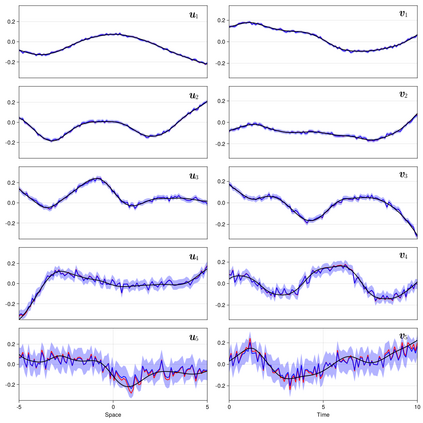
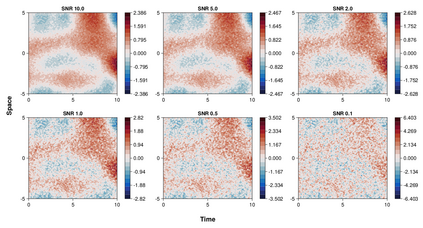
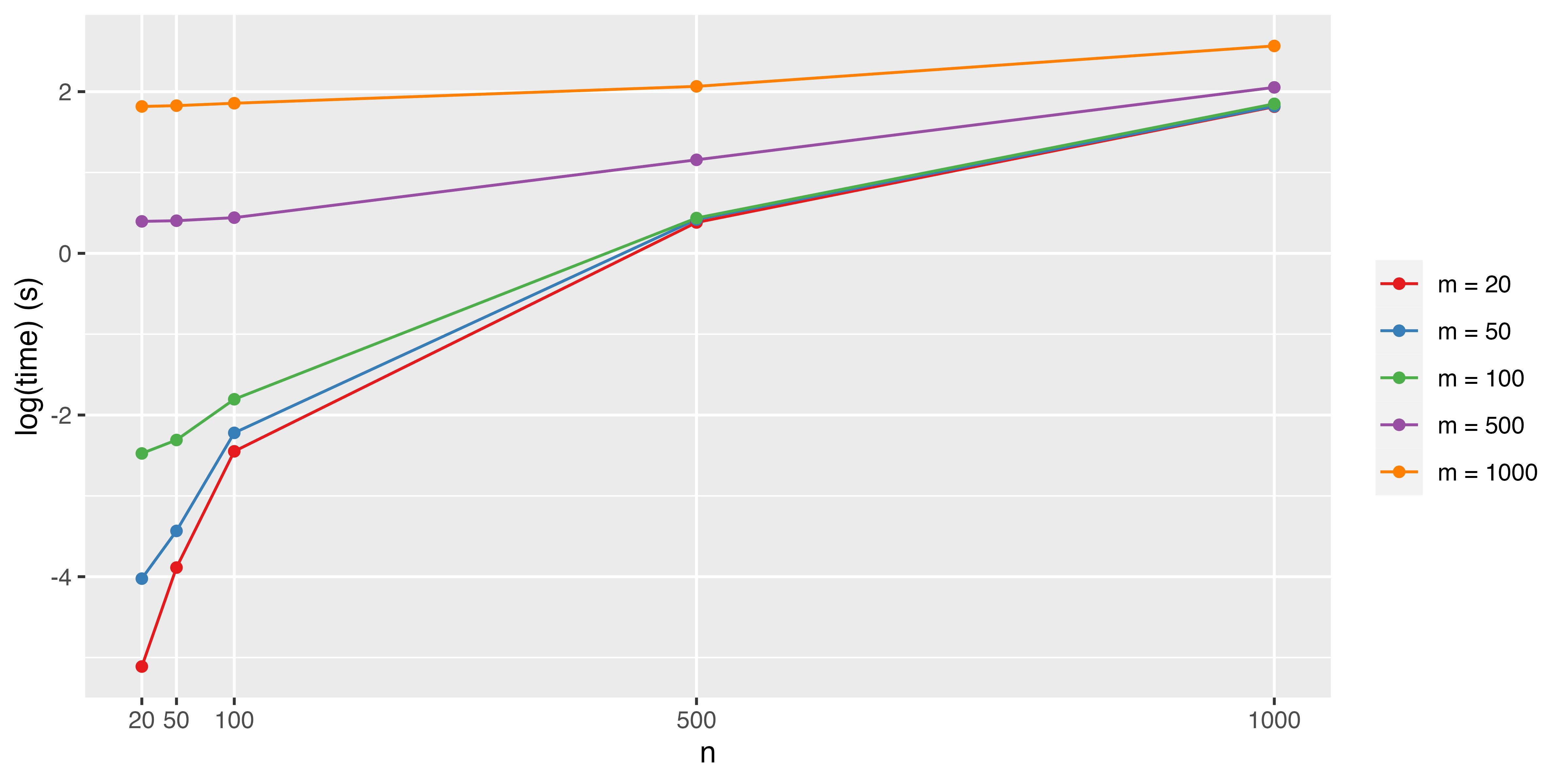
The big data era of science and technology motivates statistical modeling of matrix-valued data using a low-rank representation that simultaneously summarizes key characteristics of the data and enables dimension reduction for data compression and storage. Low-rank representations such as singular value decomposition factor the original data into the product of orthonormal basis functions and weights, where each basis function represents an independent feature of the data. However, the basis functions in these factorizations are typically computed using algorithmic methods that cannot quantify uncertainty or account for explicit structure beyond what is implicitly specified via data correlation. We propose a flexible prior distribution for orthonormal matrices that can explicitly model structure in the basis functions. The prior is used within a general probabilistic model for singular value decomposition to conduct posterior inference on the basis functions while accounting for measurement error and fixed effects. To contextualize the proposed prior and model, we discuss how the prior specification can be used for various scenarios and relate the model to its deterministic counterpart. We demonstrate favorable model properties through synthetic data examples and apply our method to sea surface temperature data from the northern Pacific, enhancing our understanding of the ocean's internal variability.