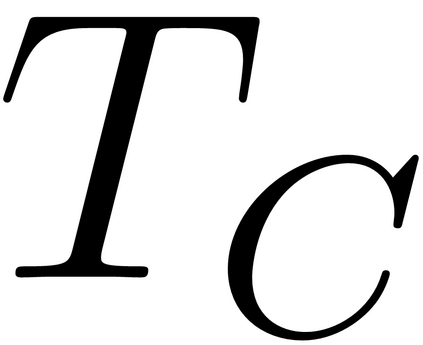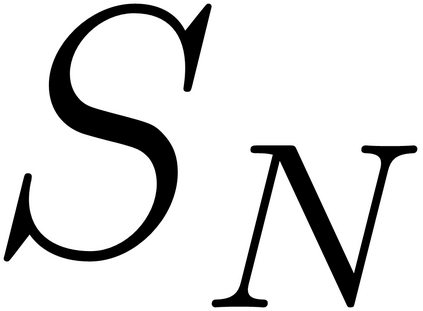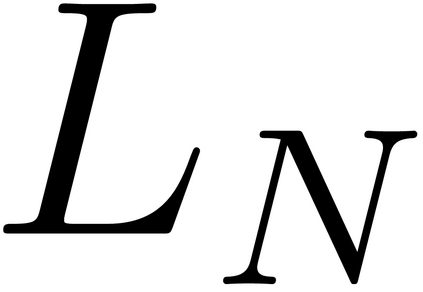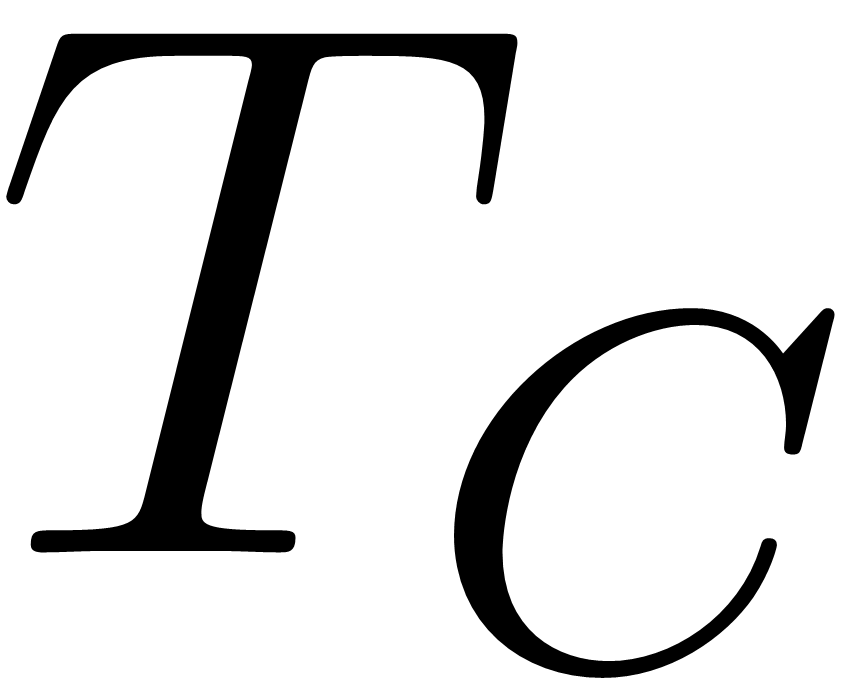Testing cross-sectional independence in panel data models is of fundamental importance in econometric analysis with high-dimensional panels. Recently, econometricians began to turn their attention to the problem in the presence of serial dependence. The existing procedure for testing cross-sectional independence with serial correlation is based on the sum of the sample cross-sectional correlations, which generally performs well when the alternative has dense cross-sectional correlations, but suffers from low power against sparse alternatives. To deal with sparse alternatives, we propose a test based on the maximum of the squared sample cross-sectional correlations. Furthermore, we propose a combined test to combine the p-values of the max based and sum based tests, which performs well under both dense and sparse alternatives. The combined test relies on the asymptotic independence of the max based and sum based test statistics, which we show rigorously. We show that the proposed max based and combined tests have attractive theoretical properties and demonstrate the superior performance via extensive simulation results. We apply the two new tests to analyze the weekly returns on the securities in the S\&P 500 index under the Fama-French three-factor model, and confirm the usefulness of the proposed combined test in detecting cross-sectional independence.
翻译:暂无翻译