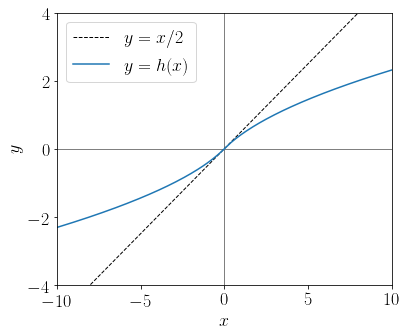
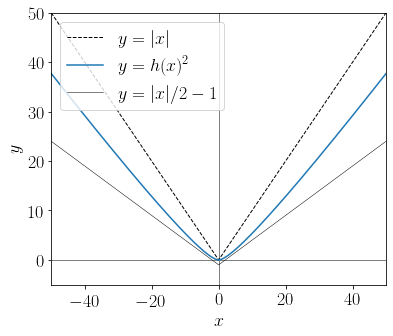
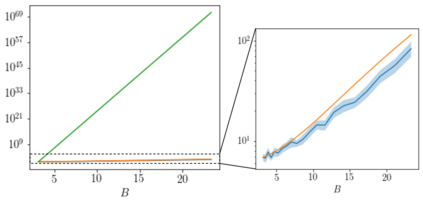
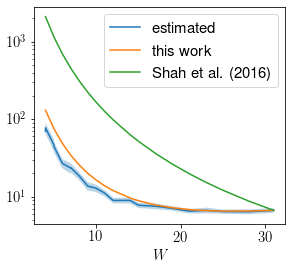
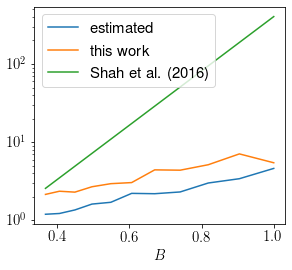
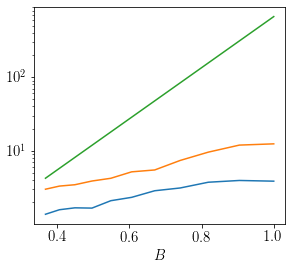
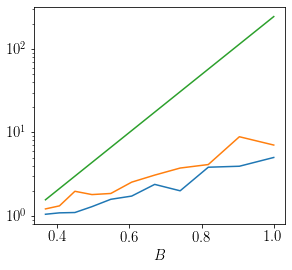
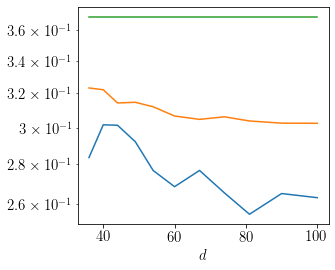
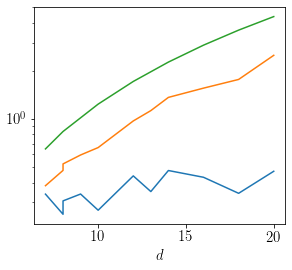
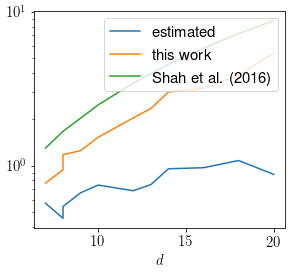
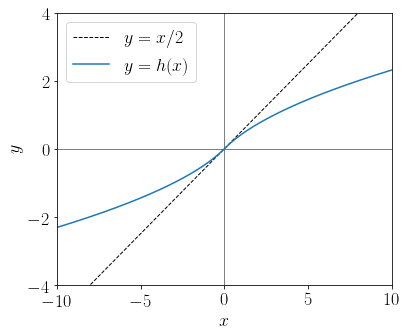
Ranking problems based on pairwise comparisons, such as those arising in online gaming, often involve a large pool of items to order. In these situations, the gap in performance between any two items can be significant, and the smallest and largest winning probabilities can be very close to zero or one. Furthermore, each item may be compared only to a subset of all the items, so that not all pairwise comparisons are observed. In this paper, we study the performance of the Bradley-Terry-Luce model for ranking from pairwise comparison data under more realistic settings than those considered in the literature so far. In particular, we allow for near-degenerate winning probabilities and arbitrary comparison designs. We obtain novel results about the existence of the maximum likelihood estimator (MLE) and the corresponding $\ell_2$ estimation error without the bounded winning probability assumption commonly used in the literature and for arbitrary comparison graph topologies. Central to our approach is the reliance on the Fisher information matrix to express the dependence on the graph topologies and the impact of the values of the winning probabilities on the estimation risk and on the conditions for the existence of the MLE. Our bounds recover existing results as special cases but are more broadly applicable.
翻译:基于对等比较(如在线游戏中产生的对等比较)的排名问题往往涉及大量需要排序的项目。在这种情况下,任何两个项目之间的性能差距可能很大,而最小和最大的赢得概率可能非常接近零或一。此外,每个项目可能只与所有项目的一个子集相比较,这样就不是所有对等比较都能够观察到。在本文中,我们研究布拉德利-Terri-Luce模型的性能,以便在比文献中迄今所考虑的更现实的环境下,从对等比较数据中进行排序。特别是,我们允许近离差性获得概率和任意比较设计。我们获得了关于存在最大可能性估计值(MLE)和相应的2美元估算误差的新结果,而没有看到文献中常用的附带的赢率假设,也没有观察到任意比较图表的顶点。我们的方法的核心是依靠渔业信息矩阵来表明对图表表的依赖性,以及赢得性概率值对估算风险和任意比较设计的影响,但对于现有案例的恢复条件具有更大的约束力。