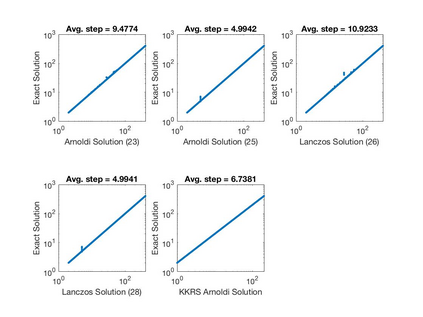
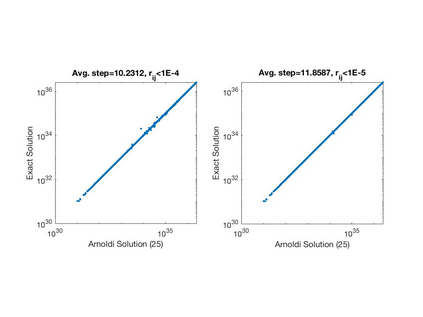
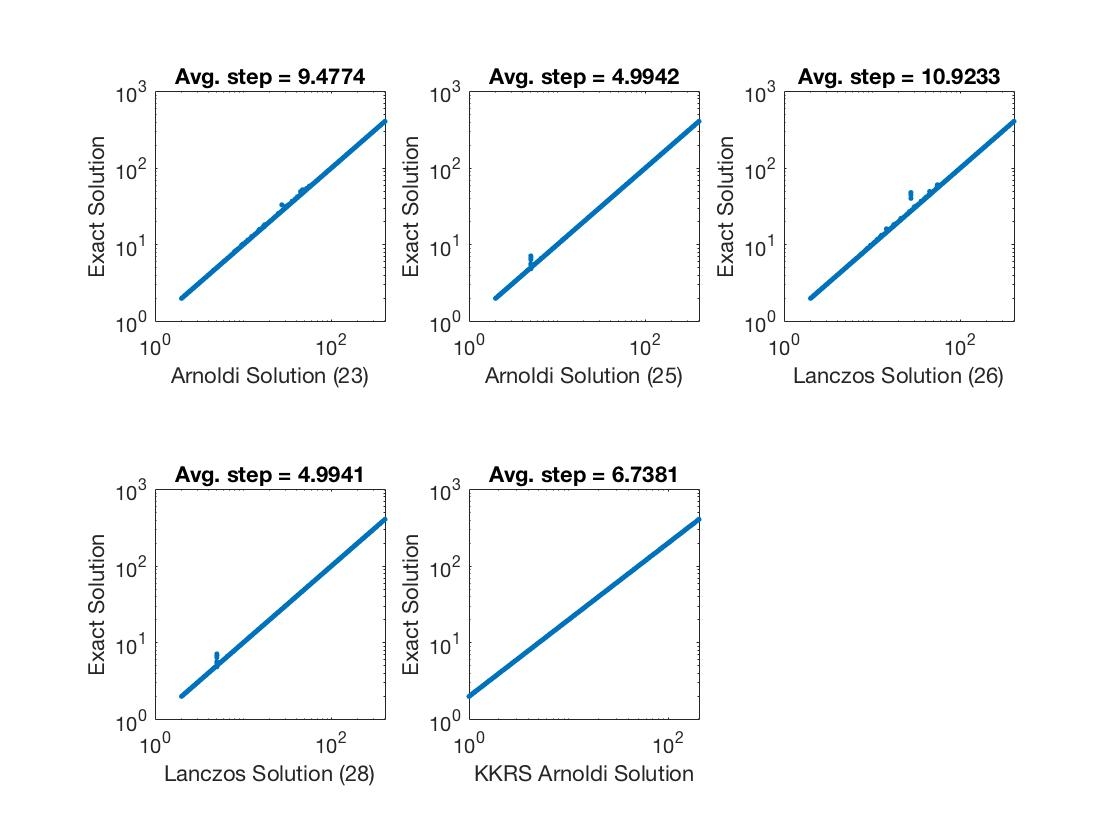
One of the properties of interest in the analysis of networks is \emph{global communicability}, i.e., how easy or difficult it is, generally, to reach nodes from other nodes by following edges. Different global communicability measures provide quantitative assessments of this property, emphasizing different aspects of the problem. This paper investigates the sensitivity of global measures of communicability to local changes. In particular, for directed, weighted networks, we study how different global measures of communicability change when the weight of a single edge is changed; or, in the unweighted case, when an edge is added or removed. The measures we study include the \emph{total network communicability}, based on the matrix exponential of the adjacency matrix, and the \emph{Perron network communicability}, defined in terms of the Perron root of the adjacency matrix and the associated left and right eigenvectors. Finding what local changes lead to the largest changes in global communicability has many potential applications, including assessing the resilience of a system to failure or attack, guidance for incremental system improvements, and studying the sensitivity of global communicability measures to errors in the network connection data.
翻译:在网络分析中,人们感兴趣的一个属性是 \ emph{ global Communicable}, 也就是说, 一般来说, 以以下边缘从其他节点达成节点是多么容易或困难。 不同的全球可交流性措施提供了对这一属性的定量评估, 强调了问题的不同方面。 本文调查了全球可交流性测量对本地变化的敏感性。 特别是, 对于定向加权网络, 我们研究当单一边缘的重量改变时, 不同的全球可交流性变化测量方法如何不同; 或者, 在未加权的情况下, 在未加分或去除边缘的情况下, 我们研究的措施包括基于相邻性矩阵矩阵矩阵指数的 \ emph{ commall communicable} 和基于相邻性矩阵根基数的 emph{ Perron 网络可交流性 。 特别是对于定向加权网络, 我们研究的是, 当单一边缘的重量改变时, 或对于未加分度最大的全球可交流性变化时, 是什么。 我们研究的措施包括基于对网络故障敏感度或攻击性系统弹性度的系统弹性、 、 数据同步性研究系统升级性 、 和递增变的系统 指南。