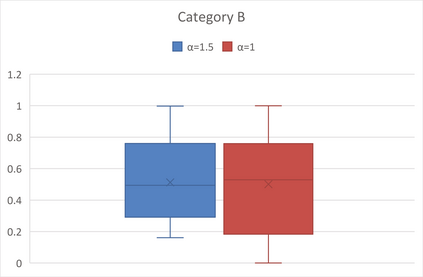
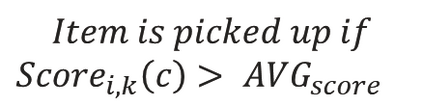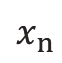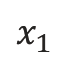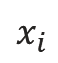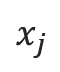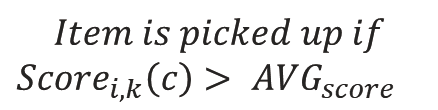The Travelling Thief Problem (TTP) is a challenging combinatorial optimization problem that attracts many scholars. The TTP interconnects two well-known NP-hard problems: the Travelling Salesman Problem (TSP) and the 0-1 Knapsack Problem (KP). Increasingly algorithms have been proposed for solving this novel problem that combines two interdependent sub-problems. In this paper, TTP is investigated theoretically and empirically. An algorithm based on the score value calculated by our proposed formulation in picking items and sorting items in the reverse order in the light of the scoring value is proposed to solve the problem. Different approaches for solving the TTP are compared and analyzed; the experimental investigations suggest that our proposed approach is very efficient in meeting or beating current state-of-the-art heuristic solutions on a comprehensive set of benchmark TTP instances.
翻译:旅行小偷问题(TTP)是一个具有挑战性的组合优化问题,它吸引了许多学者。TTP连接了两个众所周知的NP-硬问题:旅行销售商问题(TSP)和0-1Knapack问题(KP)。为解决这一新颖问题,提出了越来越多的算法,将两个相互依存的子问题结合在一起。本文对TTP进行了理论和经验方面的调查。根据我们提议的提法在根据评分值选择项目和按倒序排序项目时计算得分值的算法,提出了解决这一问题的建议。对解决TTP的不同方法进行了比较和分析;实验性调查表明,我们所提议的方法非常高效地在一套全面的基准TTP实例上应对或击败目前最先进的超理论解决办法。