Double-cover-based analysis of the Bethe permanent of non-negative matrices

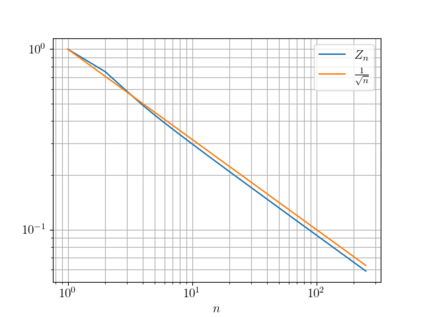
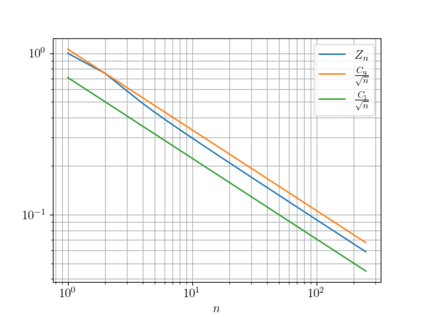
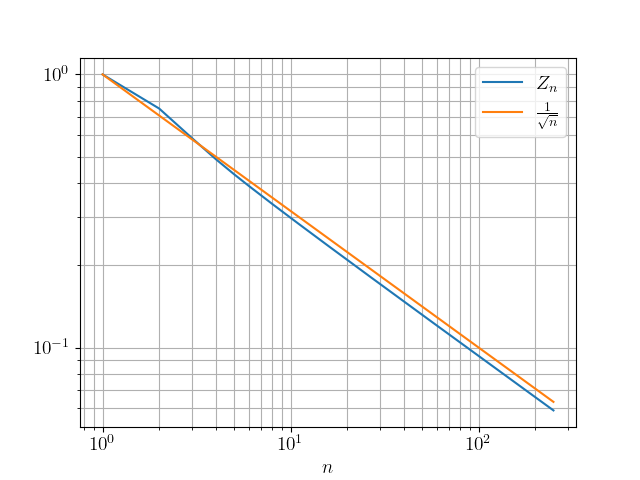
The permanent of a non-negative matrix appears naturally in many information processing scenarios. Because of the intractability of the permanent beyond small matrices, various approximation techniques have been developed in the past. In this paper, we study the Bethe approximation of the permanent and add to the body of literature showing that this approximation is very well behaved in many respects. Our main technical tool are topological double covers of the normal factor graph whose partition function equals the permanent of interest, along with a transformation of these double covers.