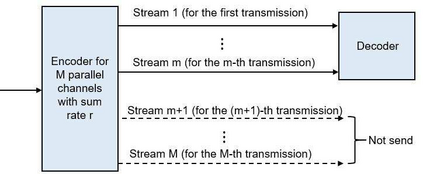
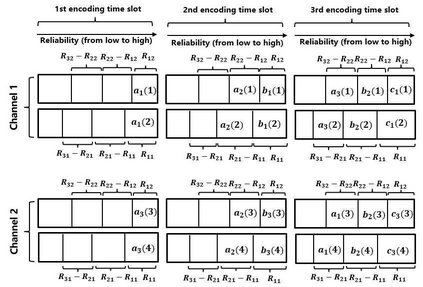
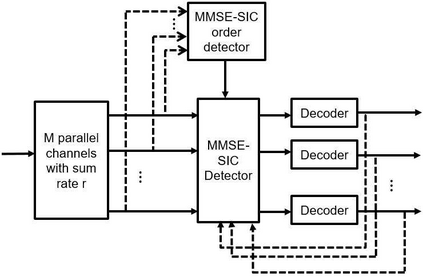
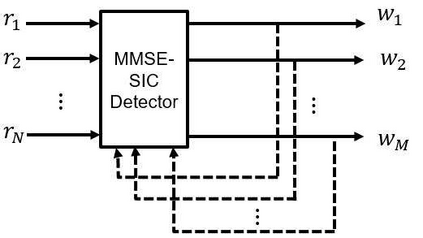
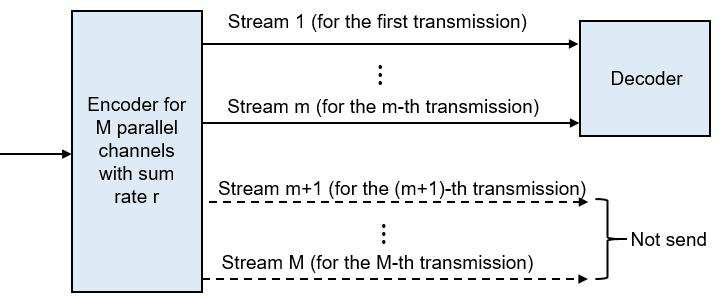
In this paper, we first propose an universal polar coding scheme for parallel Gaussian channels with non-binary inputs. It is assumed that the encoder knows only the sum capacity of M parallel channels instead of the capacity of any single channel. By decomposing each parallel channel into T = [log2r] sub channels, we therefore obtain MT binary sub-channels. A super polar coding scheme that across all sub-channels is then proposed. This scheme can achieve the sum capacity when the block length is sufficiently large. We have also discussed the applications of parallel polar coding design for both the HARQ and MIMO systems. It is shown that a capacity-achieving HARQ scheme can be obtained for block fading channel and a capacity-achieving MIMO design that requires only the feedback of the sum rate of all MIMO layers can also be attained.
翻译:在本文中,我们首先为平行高斯海峡和非二元投入的平行频道提出一个通用极地编码计划,假定编码器只知道M平行频道的总容量,而不是任何单一频道的容量。通过将每个平行频道分解为T = [log2r] 子频道,我们因此获得MT二进通道。然后提议在所有小通道之间建立一个超级极地编码计划。当块长度足够大时,这个计划可以达到总容量。我们还讨论了HARQ和MIMO系统平行极地编码设计的应用。已经表明,能够为块状淡化通道和只要求所有MIMO层总速回馈的MIMO设计实现能力达到HARQ计划,并且能够实现只要求所有MIMO层总和率的MIMO设计。