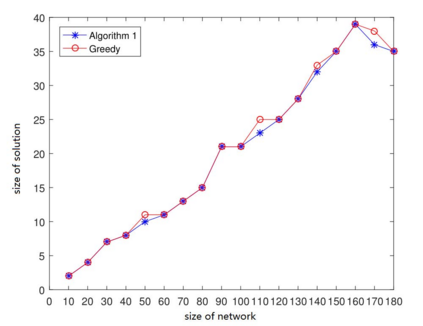
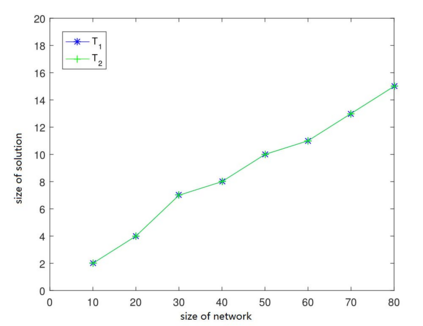
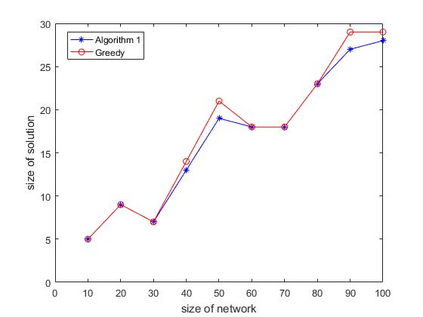
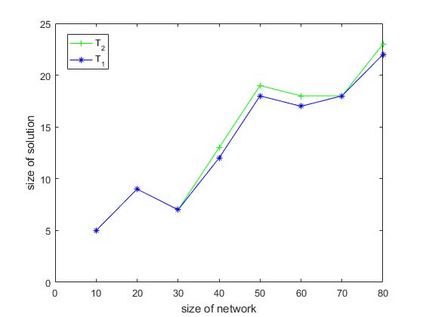
A connected dominating set is a widely adopted model for the virtual backbone of a wireless sensor network. In this paper, we design an evolutionary algorithm for the minimum connected dominating set problem (MinCDS), whose performance is theoretically guaranteed in terms of both computation time and approximation ratio. Given a connected graph $G=(V,E)$, a connected dominating set (CDS) is a subset $C\subseteq V$ such that every vertex in $V\setminus C$ has a neighbor in $C$, and the subgraph of $G$ induced by $C$ is connected. The goal of MinCDS is to find a CDS of $G$ with the minimum cardinality. We show that our evolutionary algorithm can find a CDS in expected $O(n^3)$ time which approximates the optimal value within factor $(2+\ln\Delta)$, where $n$ and $\Delta$ are the number of vertices and the maximum degree of graph $G$, respectively.
翻译:连接主导器是无线传感器网络虚拟主干网的一种广泛采用的模式。 在本文中,我们为最小连接主导器问题设计了一种演进算法(MincDS),其性能在计算时间和近似率两方面都得到理论上的保证。如果有一个连接的图形$G=(V,E),连接的主导器(CDS)是一个子数 $C\ secondseque V$,这样每根V\setminus C$的顶端都有一个以美元为单位的邻居,而每根G$的子图则是以美元为单位的。 MincDS的目标是在最小基数中找到一个以美元为单位的CDS。我们表明,我们的演进算法可以在预期的$O(n)3时间中找到CDS,该时间接近系数$(2 ⁇ \Delta)内的最佳值,其中美元和美元分别为odg $($)内的最佳值。