Empirical likelihood and uniform convergence rates for dyadic kernel density estimation

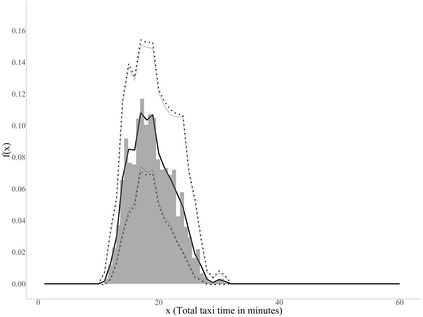
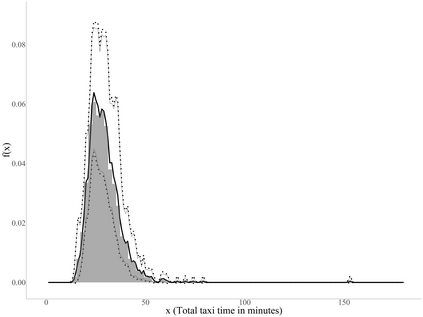
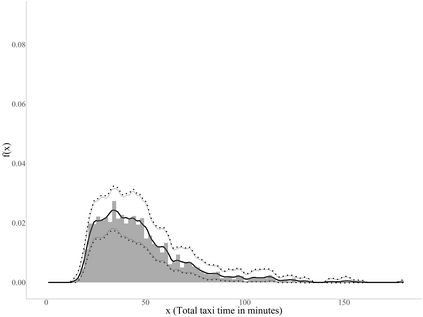
This paper studies the asymptotic properties of and alternative inference methods for kernel density estimation (KDE) for dyadic data. We first establish uniform convergence rates for dyadic KDE. Secondly, we propose a modified jackknife empirical likelihood procedure for inference. The proposed test statistic is asymptotically pivotal regardless of presence of dyadic clustering. The results are further extended to cover the practically relevant case of incomplete dyadic data. Simulations show that this modified jackknife empirical likelihood-based inference procedure delivers precise coverage probabilities even with modest sample sizes and with incomplete dyadic data. Finally, we illustrate the method by studying airport congestion in the United States.