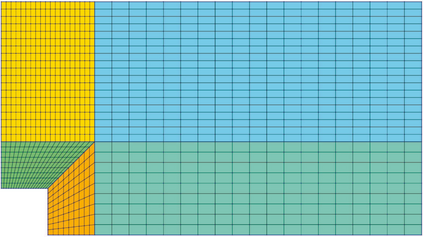
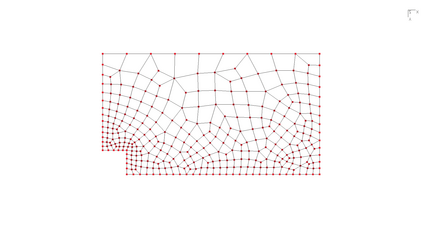
Achieving accurate numerical results of hydrodynamic loads based on the potential-flow theory is very challenging for structures with sharp edges, due to the singular behavior of the local-flow velocities. In this paper, we introduce the Extended Finite Element Method (XFEM) to solve fluid-structure interaction problems involving sharp edges on structures. Four different FEM solvers, including conventional linear and quadratic FEMs as well as their corresponding XFEM versions with local enrichment by singular basis functions at sharp edges, are implemented and compared. To demonstrate the accuracy and efficiency of the XFEMs, a thin flat plate in an infinite fluid domain and a forced heaving rectangle at the free surface, both in two dimensions, will be studied. For the flat plate, the mesh convergence studies are carried out for both the velocity potential in the fluid domain and the added mass, and the XFEMs show apparent advantages thanks to their local enhancement at the sharp edges. Three different enrichment strategies are also compared, and suggestions will be made for the practical implementation of the XFEM. For the forced heaving rectangle, the linear and 2nd order mean wave loads are studied. Our results confirm the previous conclusion in the literature that it is not difficult for a conventional numerical model to obtain convergent results for added mass and damping coefficients. However, when the 2nd order mean wave loads requiring the computation of velocity components are calculated via direct pressure integration, it takes a tremendously large number of elements for the conventional FEMs to get convergent results. On the contrary, the numerical results of XFEMs converge rapidly even with very coarse meshes, especially for the quadratic XFEM.
翻译:基于潜在流流理论的流体力负载的精确数字结果对于具有尖锐边缘的结构来说非常困难。 由于本地流速的奇特行为, 实现水力负荷的精确数字结果对于具有尖锐边缘的结构来说非常困难。 在本文中, 我们引入了扩展极极分元素法( XFEM ) 来解决涉及结构尖锐边缘的流体结构互动问题。 四个不同的 FEM 解答器, 包括传统线性和四面形FEM 以及它们相应的 XFEM 版本, 在尖锐边缘以单基函数进行本地浓缩。 为了显示 XFEM 的准确性和效率, 一个在无限流流域的薄扁平板, 以及一个在自由表面( 两个层面)的强迫的常规渐变渐变平板。 对于平板, 网状组合的趋同值研究显示由于在尖边缘的本地增强, 三个不同的浓缩战略也得到了比较, 并且将会为 XFEM 的实际执行提出建议。 对于在自由表面的磁盘中, 的硬直向直角平方形平方平方块的平方块, 直线和直径直径的递值结果会确认前平流结果。