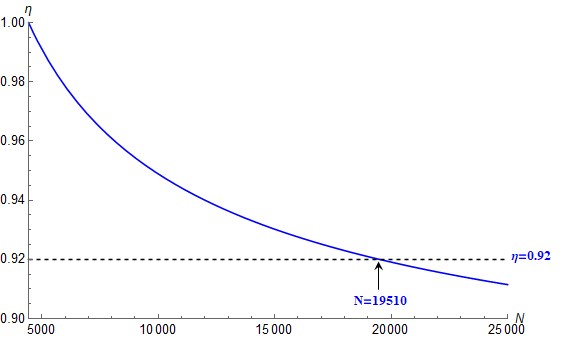
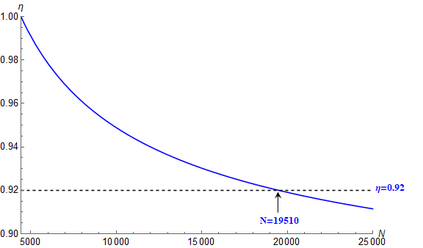
We present a device independently secure quantum scheme for p-threshold all-or-nothing oblivious transfer. Novelty of the scheme is that, its security does not depend -- unlike the usual case -- on any quantum bit commitment protocol, rather it depends on Hardy's argument for two-qubit system. This scheme is shown to be unconditionally secure against any strategy allowed by quantum mechanics. By providing a secure scheme for all-or-nothing quantum oblivious transfer, we have answered a long standing open problem, other than the quantum key distribution, whether there is any two-party quantum cryptographic protocol, which is unconditionally secure.
翻译:我们提出了一个独立安全的量子计划,用于 p-sresold all-or- nothing 无形转移。这个计划的新颖之处在于,它的可靠性并不取决于任何量比承诺协议 -- -- 与通常情况不同 -- -- 而不是取决于任何量比承诺协议,而是取决于Hardy关于二Q的论据。这个计划被证明是无条件安全的,不受量比力机制所允许的任何战略的制约。我们通过为全量或零量不显眼的转让提供一种安全计划,解决了一个长期存在的未决问题,除了量比键分配之外,我们还回答了一个长期存在的未决问题,即是否有两方的量比加密协议,这是无条件安全的。